b. 重庆大学重庆市冶金工程重点实验室, 重庆 400044;
c. 重庆大学化学化工学院, 重庆 400044
b. Chongqing Provincial Key Laboratory of Metallurgical Engineering, Chongqing University, Chongqing 400044, China;
c. School of Chemistry and Chemical Engineering, Chongqing University, Chongqing 400044, China
冶金工程中许多基本过程都会涉及一维扩散,较典型的例子有:层状液、液相界面两侧各组分的扩散[1];钢液精炼时,喷入钢液中的粉粒内的传质[1];脱气液滴内传质[2];扩散系数的实验确定涉及的扩散实验[3];金属氧化物的气体还原;金属的氧化;矿石的焙烧;金属化合物和石灰石的分解;含灰分煤的燃烧[2];气体和多孔的铁矿球团反应时,气体通过球团内部多孔固体的扩散[1, 4-8]等。
工程上涉及的这些一维扩散都是发生在有限长度区间内。而且,这些扩散速率问题基本上应该沿有明确分析结果的方向来研究。铁矿团块、钢液内每个粉粒、液滴或气泡等内部涉及的扩散或层状液、液相界面两侧各组分的扩散都是在比装置更小的尺度上描述反应器内传质问题。从反应器整体来讲,这些传质可以看作发生在装置内某局部区域,甚至某位置。当对整个反应器内流动与混合、热和质量传输、化学反应等进行综合数值模拟研究的时候,一般要求装置内更小尺度上的传质速率、反应速率等最好有明确的数学分析式[9-13]。若装置内各个更小尺度上的(或某位置上的)传质速率等也需像整个装置的传输问题处理一样,采用数值的方法迭代计算,这样势必增大问题的复杂性,降低处理问题的效率。
但一维扩散方程在无限长度区间[0,∞]或[-∞,∞]有分析解;其一是误差函数解[1, 3, 14-15],其二是高斯解[3]。在[a,b]有限长度的区间里,除了无穷级数[1, 3, 14-15]解之外,没有其他形式的分析解。无穷级数解利用起来“很不方便”[14],一般将其制成线图使用且最终利用结果也是近似值,文献[14]对级数解的推导等有详细的叙述。由于对质量守恒原理、扩散物理本质体现得不明显、不直接,导出误差解、高斯解和级数解等所用的分离变量等纯数学方法工程上很少应用。寻求有限长度区间[a,b]上扩散方程的近似解,可避免对误差函数解的依赖(即要获得物质由此位置经有限距离向彼位置扩散过程的解时,可以避免将彼位置处理在无穷远处),也可提供除级数解之外的分析利用途径,不仅具有理论意义,也是现实实践的需要。
适应工程研究和实践应用扩散方程的前述发展方向,笔者曾获得扩散方程的一个稳态近似解[16],但作为动力学的一种研究方法——稳态近似法的发展,很多内容还需要进一步阐述和讨论。对稳态假设内容的发展需要更合理的阐述;由于非稳态问题的结果是随时间变化的,因此不能利用稳态假设最后将之处理成与时间无关的结果,稳态假设的利用程度需要明确,即在扩散方程的稳态近似分析中,要明确利用稳态假设仅是获得各步骤传质速率的近似表达式;稳态近似解还需要更充分的讨论。
适应工程研究和实践应用扩散方程的前述发展方向,笔者将直接基于物质守恒原理、菲克扩散定律等,利用稳态近似法获得二、三类边界条件下非稳态扩散方程在有限长度区间上的分析解,并将近似分析解和有足够精确度的数值解做了对比。较满意结果的获得,进一步验证了笔者对稳态近似法发展的合理性。
1 处理非稳态问题的稳态近似法笔者获得的近似解是基于稳态近似法。它是处理连串反应动力学问题的一种方法[17]。对连串反应:A→R→C。为了推得A生成最终产物C的速率方程,假设中间产物R的浓度cR=const(或

|
图 1 串联反应传质过程[1] |
在获得其综合反应速率式时,可假设各环节的速率互相相等,即假设rAB=rBC=rCD=rDE=rEF=…;或也可假设B、C、D、E、F等各处的浓度随时间不变,即假设cB=const,cC=const,cD=const,cE=const,cF=const,…。未反应核模型,就是在产物层还原物质浓度c=const的稳态假设下推出的[1, 3];金属氧化反应速率是在假设氧化膜内氧化气体浓度不随时间变化的前提下得到的[18]。
由物质守恒定理可知:各环节的速率相同,就相当于所有中间物质的浓度恒定;中间各物质的浓度不随时间而改变,各环节的速率必相等。因此,中间物质浓度恒定的假设和各环节速率互相相等的假设是等价的[1]。宏观动力学中各类动力学方程(如液、液反应的双膜理论,气、固反应的各种模型)的推导均采用此两类传统的等价稳态假设内容。
在图 1所示的串联传质过程中,假设各环节的速率互相相等,亦即假设rAB-rBC=0,rBC-rCD=0,rCD-rDE=0,rDE-rEF=0,…。笔者对扩散方程的稳态近似法处理,将以发展后的稳态假设内容为基础。同样对图 1所示的传质过程,由物质守恒可知:若各环节的速率不一定互相相等,所有中间物质的浓度不一定恒定;但若rAB-rBC=const,rBC-rCD=const,rCD-rDE= const,rDE-rEF= const,…,所有中间物质浓度的变化率(浓度对时间的导数)恒定;若中间各物质的浓度变化率不随时间而改变,各环节的速率必满足rAB-rBC=const,rBC-rCD= const,rCD-rDE= const,rDE-rEF= const,…。因此利用稳态近似法研究非稳态问题时,可进行rAB-rBC=const,rBC-rCD= const,rCD-rDE= const,rDE-rEF= const,…的假设,或进行中间物质浓度的变化率随时间不变的假设(
容易看出:传统的浓度随时间不变的假设(ci=const,i=B,C,D,E,F,…)是浓度变化率随时间不变假设(
上述对稳态假设内容的合理发展,减轻了稳态假设的约束。笔者认为:稳态假设就是在由诸多串行环节组成的非稳态问题的处理过程中,为获得各环节传质(或反应)速率或总传质速率而附加的某个(些)量随时间不变的有限约束。浓度随时间不变的假设或浓度变化率随时间不变的假设,都是处理非稳态问题而附加的某(些)量随时间不变的稳态约束;浓度随时间不变、浓度变化率随时间不变都是相对非稳态问题而说的,它们都是稳态假设。把非稳态问题中理应随时间变化的浓度假设为不变的叫稳态假设,那么把非稳态问题中理应随时间变化的浓度变化率假设为不变的也应该叫稳态假设。应用浓度变化率随时间不变假设获得动力学方程时,可以参照利用浓度随时间不变假设获得双膜理论、未反应核模型等所采用的推导过程。
看如何利用
| $ \frac{{\partial c}}{{\partial t}} = D\frac{{{\partial ^2}c}}{{\partial {x^2}}}, $ | (1) |
初始条件t=0, x∈(0, l), c=0;
边界条件t>0,x=0, D
上述非稳态问题的解主要就是获得(0,l)内任一位置x的浓度cx(t)随时间变化的关系,见图 2,位置x处的浓度cx(t)只是时间t的函数。运用稳态近似法只能求得cx(t)的近似值cx。求cx的步骤如下:

|
图 2 扩散方程解的图示 |
求cx的稳态近似法以数值方法的原理为基础,首先需要合适的数值网格。将以位置x为中心、长度为Δx的区间作为浓度cx所在的控制容积。将位于区间Δx左边的区间长度x-0.5Δx均分为n-1份,则每一份的长度是
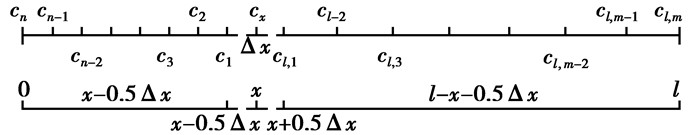
|
图 3 非稳态扩散方程离散用图 |
每个间隔位置处的浓度都有自己的控制容积,浓度c1的控制容积为
| $ D\frac{{{c_2} - {c_1}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} - D\frac{{{c_1} - {c_{l,1}}}}{{\Delta x}} = \frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}}\frac{1}{2}\left( {\frac{{x - 0.5\Delta x}}{{n - 1}} + \Delta x} \right)。$ | (2-1) |
注意,这里与一般数值方法不一样的是[19]:浓度变化率(浓度对时间的导数)不离散,仅是关于对位置的导数的项离散;因c1是x-0.5Δx处的浓度,它只是时间的函数,所以在c1控制容积内,
| $ D\frac{{{c_3} - {c_2}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} - D\frac{{{c_2} - {c_1}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} = \frac{{{\rm{d}}{c_2}}}{{{\rm{d}}t}}\frac{{x - 0.5\Delta x}}{{n - 1}}, $ | (2-2) |
| $ D\frac{{{c_4} - {c_3}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} - D\frac{{{c_3} - {c_2}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} = \frac{{{\rm{d}}{c_3}}}{{{\rm{d}}t}}\frac{{x - 0.5\Delta x}}{{n - 1}}, $ | (2-3) |
| $ \begin{array}{*{20}{c}} { \cdots \cdots }\\ {D\frac{{{c_i} - {c_{i - 1}}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} - D\frac{{{c_{i - 1}} - {c_{i - 2}}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} = \frac{{{\rm{d}}{c_{i - 1}}}}{{{\rm{d}}t}}\frac{{x - 0.5\Delta x}}{{n - 1}},} \end{array}\;\;\;\;\;\;\;\;\;\;\left[ {2 - \left( {i - 1} \right)} \right] $ |
| $ \begin{array}{*{20}{c}} { \cdots \cdots }\\ {D\frac{{{c_n} - {c_{n - 1}}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} - D\frac{{{c_{n - 1}} - {c_{n - 2}}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} = \frac{{{\rm{d}}{c_{n - 1}}}}{{{\rm{d}}t}}\frac{{x - 0.5\Delta x}}{{n - 1}},} \end{array}\;\;\;\;\;\;\;\;\;\;\left[ {2 - \left( {n - 1} \right)} \right] $ |
左边界的边界条件D
| $ \beta \left( {{c^0} - {c_n}} \right) - D\frac{{{c_n} - {c_{n - 1}}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} = 0。\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\left( {2 - n} \right) $ |
对cl,1、c l,2,…,c l,m-2的控制容积,扩散方程分别离散为
| $ D\frac{{{c_1} - {c_{l,1}}}}{{\Delta x}} - D\frac{{{c_{l,1}} - {c_{l,2}}}}{{\frac{{l - x - 0.5\Delta x}}{{m - 1}}}} = \frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}}\frac{1}{2}\left( {\frac{{l - x - 0.5\Delta x}}{{m - 1}} + \Delta x} \right), $ | (3-1) |
| $ D\frac{{{c_{l,1}} - {c_{l,2}}}}{{\frac{{l - x - 0.5\Delta x}}{{m - 1}}}} - D\frac{{{c_{l,2}} - {c_{l,3}}}}{{\frac{{l - x - 0.5\Delta x}}{{m - 1}}}} = \frac{{{\rm{d}}{c_{l,2}}}}{{{\rm{d}}t}}\frac{{l - x - 0.5\Delta x}}{{m - 1}}, $ | (3-2) |
| $ \begin{array}{*{20}{c}} { \cdots \cdots }\\ {D\frac{{{c_{l,m - 3}} - {c_{l,m - 2}}}}{{\frac{{l - x - 0.5\Delta x}}{{m - 1}}}} - D\frac{{{c_{l,m - 2}} - {c_{l,m - 1}}}}{{\frac{{l - x - 0.5\Delta x}}{{m - 1}}}} = \frac{{{\rm{d}}{c_{l,m - 2}}}}{{{\rm{d}}t}}\frac{{l - x - 0.5\Delta x}}{{m - 1}}。} \end{array}\;\;\;\;\;\;\;\;\;\left[ {3 - \left( {m - 2} \right)} \right] $ |
x=l处的边界条件
| $ \frac{{{c_{l,m - 1}} - {c_{l,m}}}}{{\frac{{l - x - 0.5\Delta x}}{{m - 1}}}} = 0, $ |
扩散方程在cl,m-1的控制容积离散为
| $ D\frac{{{c_{l,m - 2}} - {c_{l,m - 1}}}}{{\frac{{l - x - 0.5\Delta x}}{{m - 1}}}} - D\frac{{{c_{l,m - 1}} - {c_{l,m}}}}{{\frac{{l - x - 0.5\Delta x}}{{m - 1}}}} = \frac{{{\rm{d}}{c_{l,m - 1}}}}{{{\rm{d}}t}}\frac{{l - x - 0.5\Delta x}}{{m - 1}}, $ |
结合右边界条件离散式,此式化为
| $ D\frac{{{c_{l,m - 2}} - {c_{l,m - 1}}}}{{\frac{{l - x - 0.5\Delta x}}{{m - 1}}}} = \frac{{{\rm{d}}{c_{l,m - 1}}}}{{{\rm{d}}t}}\frac{{l - x - 0.5\Delta x}}{{m - 1}}。\;\;\;\;\;\;\;\left[ {3 - \left( {m - 1} \right)} \right] $ |
c1,c2,c3,…,cn-1,cl,1,c l,2,c l,3,…,c l,m-1的控制容积之和连续覆盖了整个计算区域(0,l),不能再针对Δx建立关于cx的离散方程。不过,当Δx足够小时,有
| $ {c_x} = \frac{{{c_1} + {c_{l,1}}}}{2}。$ |
因此要获得浓度cx随时间变化的关系式,需要获得c1、cl,1随时间变化的关系式。在式(2-1) 和式(3-1) 中,令扩散速率
液、液反应的双膜理论总反应速率及其各步骤速率、气、固反应的各种模型(如未反应核模型)的总反应速率及其各步骤速率都是在稳态假设下获得的。利用稳态近似法可以获得非稳态问题各步骤的速率。扩散速率J、Jl的表达式也可由稳态假设获得。
式(1) 按图 3网格离散后,物理上就形成了如图 1所示的一个系列串联的传质过程。可作假设如下:
| $ \begin{array}{l} \frac{{{\rm{d}}{c_{n - 1}}}}{{{\rm{d}}t}} = {\rm{cons}}t,\frac{{{\rm{d}}{c_{n - 2}}}}{{{\rm{d}}t}} = {\rm{cons}}t, \cdots ,\frac{{{\rm{d}}{c_2}}}{{{\rm{d}}t}} = {\rm{cons}}t,\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} = {\rm{cons}}t,\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} = {\rm{cons}}t,\frac{{{\rm{d}}{c_{l,2}}}}{{{\rm{d}}t}} = \\{\rm{cons}}t, \cdots ,\frac{{{\rm{d}}{c_{l,m - 2}}}}{{{\rm{d}}t}} = {\rm{cons}}t,\\ \frac{{{\rm{d}}{c_{l,m - 1}}}}{{{\rm{d}}t}} = {\rm{cons}}t。\end{array} $ |
这也相当于假设:
| $ D\frac{{{c_n} - {c_{n - 1}}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} - D\frac{{{c_{n - 1}} - {c_{n - 2}}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} = {\rm{cons}}t, \cdots ,D\frac{{{c_2} - {c_1}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} - D\frac{{{c_1} - {c_{l,1}}}}{{\Delta x}} = {\rm{cons}}t; $ |
| $ D\frac{{{c_1} - {c_{l,1}}}}{{\Delta x}} - D\frac{{{c_{l,1}} - {c_{l,2}}}}{{\frac{{l - x - 0.5\Delta x}}{{m - 1}}}} = {\rm{cons}}t, \cdots ,D\frac{{{c_{l,m - 2}} - {c_{l,m - 1}}}}{{\frac{{l - x - 0.5\Delta x}}{{m - 1}}}} = {\rm{cons}}t。$ |
依据这些假设可以确定每步骤的传质量与时间的近似函数关系,以下为扩散速率J、Jl的确定。
由假设可推得浓度ci(5≤i≤n)的通式为
| $ \begin{array}{*{20}{c}} {{c_i} = {A_i}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^2}\frac{1}{D}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {B_i}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - }\\ {{B_i}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + \frac{{{C_i}}}{2}\frac{1}{D}\left( {\frac{{x - 0.5\Delta x}}{{n - 1}} + \Delta x} \right)\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {c_1} + }\\ {{C_i}\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_1} - {C_i}\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_{l,1}}。} \end{array} $ | (4) |
其中:
令式(2-2)+式(2-3)+式(2-4)+…+式(2-n)得:
| $ \begin{array}{*{20}{c}} {\beta \left( {{c^0} - {c_n}} \right) - D\frac{{{c_2} - {c_1}}}{{\frac{{x - 0.5\Delta x}}{{n - 1}}}} = \frac{{{\rm{d}}{c_2}}}{{{\rm{d}}t}}\frac{{x - 0.5\Delta x}}{{n - 1}} + \frac{{{\rm{d}}{c_3}}}{{{\rm{d}}t}}\frac{{x - 0.5\Delta x}}{{n - 1}} \cdots + }\\ {\frac{{{\rm{d}}{c_{i - 1}}}}{{{\rm{d}}t}}\frac{{x - 0.5\Delta x}}{{n - 1}} \cdots + \frac{{{\rm{d}}{c_{n - 1}}}}{{{\rm{d}}t}}\frac{{x - 0.5\Delta x}}{{n - 1}},} \end{array} $ |
所以
| $ J = \beta \left( {{c^0} - {c_n}} \right) - \frac{{x - 0.5\Delta x}}{{n - 1}}\left( {\frac{{{\rm{d}}{c_2}}}{{{\rm{d}}t}} + \frac{{{\rm{d}}{c_3}}}{{{\rm{d}}t}} + \frac{{{\rm{d}}{c_4}}}{{{\rm{d}}t}} + \cdots \frac{{{\rm{d}}{c_{i - 1}}}}{{{\rm{d}}t}} + \cdots \frac{{{\rm{d}}{c_{n - 1}}}}{{{\rm{d}}t}}} \right)。$ |
在式(4) 中,令i=n,得cn的表达式;令i= n-1,得cn-1的表达式,从而可得
| $ \begin{array}{*{20}{c}} {J = \beta \left[ {{c^0} - \frac{{\left( {n - 2} \right)\left( {n - 1} \right)}}{2}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^2}\frac{1}{D}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - \frac{{\left( {n - 2} \right)\left( {n - 1} \right)n}}{6}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + } \right.}\\ {\frac{{\left( {n - 2} \right)\left( {n - 1} \right)n}}{6}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} - \frac{{n - 1}}{2}\frac{1}{D}\left( {\frac{{x - 0.5\Delta x}}{{n - 1}} + \Delta x} \right)\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - }\\ {\left. {{c_1} - \left( {x - 0.5\Delta x} \right)\frac{1}{{\Delta x}}{c_1} + \left( {x - 0.5\Delta x} \right)\frac{1}{{\Delta x}}{c_{l,1}}} \right] - \frac{{x - 0.5\Delta x}}{{n - 1}}\left( {n - 2} \right)\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - }\\ {{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^2}\frac{{\left( {n - 2} \right)\left( {n - 1} \right)}}{2}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^2}\frac{{\left( {n - 2} \right)\left( {n - 1} \right)}}{2}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}}。} \end{array} $ | (5) |
按照同样的做法,由式(3-1) 至式[3-(m-2)]可以推得cl,i的表达式为
| $ \begin{array}{*{20}{c}} {{c_{l,i}} = {A_i}{{\left( {\frac{{l - x - 0.5\Delta x}}{{m - 1}}} \right)}^2}\frac{1}{D}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + {B_i}{{\left( {\frac{{l - x - 0.5\Delta x}}{{m - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} - }\\ {{B_i}{{\left( {\frac{{l - x - 0.5\Delta x}}{{m - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + \frac{{{C_i}}}{2}\frac{1}{D}\left( {\frac{{l - x - 0.5\Delta x}}{{m - 1}} + \Delta x} \right)\frac{{l - x - 0.5\Delta x}}{{m - 1}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + {c_{l,1}} + }\\ {{C_i}\frac{{l - x - 0.5\Delta x}}{{m - 1}}\frac{1}{{\Delta x}}{c_{l,1}} - {C_i}\frac{{l - x - 0.5\Delta x}}{{m - 1}}\frac{1}{{\Delta x}}{c_1}。} \end{array} $ |
其中,5≤i≤m-1;
令式(3-2)+式(3-3)+式(3-4)+…+式[3-(m-1)]得:
| $ D\frac{{{c_{l,1}} - {c_{l,2}}}}{{\frac{{l - x - 0.5\Delta x}}{{m - 1}}}} = \frac{{{\rm{d}}{c_{l,2}}}}{{{\rm{d}}t}}\frac{{l - x - 0.5\Delta x}}{{m - 1}} + \cdots + \frac{{{\rm{d}}{c_{l,m - 2}}}}{{{\rm{d}}t}}\frac{{l - x - 0.5\Delta x}}{{m - 1}} + \frac{{{\rm{d}}{c_{l,m - 1}}}}{{{\rm{d}}t}}\frac{{l - x - 0.5\Delta x}}{{m - 1}} $ |
所以得Jl与
| $ \begin{array}{*{20}{c}} {{J_l} = \frac{{l - x - 0.5\Delta x}}{{m - 1}}\left( {m - 2} \right)\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + {{\left( {\frac{{l - x - 0.5\Delta x}}{{m - 1}}} \right)}^2}\frac{{\left( {m - 2} \right)\left( {m - 1} \right)}}{2}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} - }\\ {{{\left( {\frac{{l - x - 0.5\Delta x}}{{m - 1}}} \right)}^2}\frac{{\left( {m - 2} \right)\left( {m - 1} \right)}}{2}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{1}}}}{{{\rm{d}}t}}。} \end{array} $ | (6) |
毫无疑问,无任何约束情况下,式(1) 的非稳态扩散方程在x-0.5Δx和x+0.5Δx处的值c1、cl,1随时间是变化的,
| $ \begin{array}{*{20}{c}} {\frac{{\left( {n - 2} \right)\left( {n - 1} \right)}}{{2{{\left( {n - 1} \right)}^2}}}{{\left( {x - 0.5\Delta x} \right)}^2}\frac{\beta }{D}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - \frac{{\left( {n - 2} \right)\left( {n - 1} \right)n}}{{6{{\left( {n - 1} \right)}^3}}}{{\left( {x - 0.5\Delta x} \right)}^3}\frac{\beta }{D}\left( {\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} - \frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}}} \right) + }\\ {\frac{\beta }{{2D}}\frac{{{{\left( {x - 0.5\Delta x} \right)}^2}}}{{n - 1}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + \frac{\beta }{{2D}}\Delta x\left( {x - 0.5\Delta x} \right)\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + \beta {c_1} - \beta \left( {x - 0.5\Delta x} \right)\left( {\frac{1}{{\Delta x}}{c_{l,1}} - \frac{1}{{\Delta x}}{c_1}} \right) + }\\ {\frac{{n - 2}}{{n - 1}}\left( {x - 0.5\Delta x} \right)\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - \frac{{\left( {n - 2} \right)\left( {n - 1} \right)}}{{2{{\left( {n - 1} \right)}^2}}}{{\left( {x - 0.5\Delta x} \right)}^2}\left( {\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} - \frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}}} \right) + \frac{1}{2}\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + }\\ {\frac{{\Delta x}}{2}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - D\left( {\frac{{{c_{l,1}}}}{{\Delta x}} - \frac{{{c_1}}}{{\Delta x}}} \right) = \beta {c^0}。} \end{array} $ | (7) |
将Jl的表达式[式(6)]代入式(3-1),整理得到c1、cl,1满足的另一个关系式:
| $ \begin{array}{*{20}{c}} {\frac{{m - 2}}{{m - 1}}\left( {l - x - 0.5\Delta x} \right)\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + \frac{{{{\left( {l - x - 0.5\Delta x} \right)}^2}}}{{\Delta x}}\frac{{\left( {m - 2} \right)}}{{2\left( {m - 1} \right)}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} - }\\ {\frac{{{{\left( {l - x - 0.5\Delta x} \right)}^2}}}{{\Delta x}}\frac{{\left( {m - 2} \right)}}{{2\left( {m - 1} \right)}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + \frac{1}{2}\left( {\frac{{l - x - 0.5\Delta x}}{{m - 1}} + \Delta x} \right)\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + \frac{D}{{\Delta x}}{c_{l,1}} - \frac{D}{{\Delta x}}{c_1} = 0。} \end{array} $ | (8) |
式(7) 和式(8) 是关于两个未知函数c1、cl,1的常系数线性微分方程组。按式(1) 的初始条件,式(7) 和式(8) 中c1、cl,1在0时刻的值为
| $ {c_1}\left| {_{t = 0}} \right. = 0, $ | (9) |
| $ {c_{l,1}}\left| {_{t = 0}} \right. = 0。$ | (10) |
由式(7)、式(8)、式(9) 及式(10) 决定的c1、cl,1就是扩散方程在x-0.5Δx和x+0.5Δx处的近似值。c1、cl,1与x-0.5Δx和x+0.5Δx处精确值的偏差来自两个方面,一方面由于采用了基于稳态假设而推导的扩散速率J、Jl的近似表达式,另一方面是因为每个离散方程对应的控制容积没有趋于零,即c1、cl,1之间的距离Δx没有趋于零,n没有趋于无穷大,m没有趋于无穷大。
2.4 导出cx满足的关系式为了消除各控制容积没有趋于零带来的偏差,令n→∞,式(7) 首先变为
| $ \begin{array}{*{20}{c}} {{{\left( {x - 0.5\Delta x} \right)}^2}\frac{\beta }{{2D}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - {{\left( {x - 0.5\Delta x} \right)}^3}\frac{\beta }{{6D}}\left( {\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} - \frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}}} \right) + \frac{\beta }{{2D}}\Delta x\left( {x - 0.5\Delta x} \right)\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + }\\ {\beta {c_1} - \beta \left( {x - 0.5\Delta x} \right)\left( {\frac{1}{{\Delta x}}{c_{l,1}} - \frac{1}{{\Delta x}}{c_1}} \right) + \left( {x - 0.5\Delta x} \right)\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - }\\ {\frac{1}{2}\left( {x - 0.5\Delta x} \right)\left( {\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} - \frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}}} \right) + \frac{{\Delta x}}{2}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - D\left( {\frac{{{c_{l,1}}}}{{\Delta x}} - \frac{{{c_1}}}{{\Delta x}}} \right) = \beta {c^0}。} \end{array} $ |
鉴于cl,1Δx-c1Δx=cl,1-c1Δx,1Δxdcl,1dt-1Δxdc1dt=dcl,1-c1Δxdt,1Δxdcl,1dt-1Δxdc1dt和cl,1Δx-c1Δx在Δx→0时的极限都为有限值,因此Δx→0时,上式可进一步化简为
| $ \begin{array}{*{20}{c}} {\left( {\frac{\beta }{{2D}}{x^2} + \frac{\beta }{{6D}}\frac{{{x^3}}}{{\Delta x}} + x + \frac{1}{2}\frac{{{x^2}}}{{\Delta x}}} \right)\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - \left( {\frac{\beta }{{6D}}\frac{{{x^3}}}{{\Delta x}} + \frac{1}{2}\frac{{{x^2}}}{{\Delta x}}} \right)\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + }\\ {\left( {\beta + \beta \frac{x}{{\Delta x}} + \frac{D}{{\Delta x}}} \right){c_1} - \left( {\frac{D}{{\Delta x}} + \beta \frac{x}{{\Delta x}}} \right){c_{l,1}} = \beta {c^0}。} \end{array} $ | (11) |
令m→∞,并考虑到Δx为无穷小,同样地式(8) 化简为
| $ \left[ {l - x + \frac{1}{2}\frac{{{{\left( {l - x} \right)}^2}}}{{\Delta x}}} \right]\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} - \frac{1}{2}\frac{{{{\left( {l - x} \right)}^2}}}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}}\frac{D}{{\Delta x}}{c_{l,1}} - \frac{D}{{\Delta x}}{c_1} = 0。$ | (12) |
式(11)×(l-x)2(2
| $ \begin{array}{*{20}{c}} {\left[ {\left( {\frac{\beta }{{2D}}{x^2} + \frac{\beta }{{6D}}\frac{{{x^3}}}{{\Delta x}} + x + \frac{1}{2}\frac{{{x^2}}}{{\Delta x}}} \right){{\left( {l - x} \right)}^2}\left( {2\frac{{\Delta x}}{{l - x}} + 1} \right) - \frac{1}{2}\frac{{{{\left( {l - x} \right)}^2}}}{{\Delta x}}{x^2}\left( {\frac{\beta }{{3D}}x + 1} \right)} \right]\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + }\\ {\left[ {\left( {\beta + \beta \frac{x}{{\Delta x}} + \frac{D}{{\Delta x}}} \right){{\left( {l - x} \right)}^2}\left( {2\frac{{\Delta x}}{{l - x}} + 1} \right) - \frac{D}{{\Delta x}}{x^2}\left( {\frac{\beta }{{3D}}x + 1} \right)} \right]{c_1} + }\\ {\left[ {\frac{D}{{\Delta x}}{x^2}\left( {\frac{\beta }{{3D}}x + 1} \right) - \left( {\frac{D}{{\Delta x}} + \beta \frac{x}{{\Delta x}}} \right){{\left( {l - x} \right)}^2}\left( {2\frac{{\Delta x}}{{l - x}} + 1} \right)} \right]{c_{l,1}} = \beta {c^0}{{\left( {l - x} \right)}^2}\left( {2\frac{{\Delta x}}{{l - x}} + 1} \right)。} \end{array} $ | (13) |
式(13) 对t求导得:
| $ \begin{array}{*{20}{c}} {\left[ {\left( {\frac{\beta }{{2D}}{x^2} + \frac{\beta }{{6D}}\frac{{{x^3}}}{{\Delta x}} + x + \frac{1}{2}\frac{{{x^2}}}{{\Delta x}}} \right){{\left( {l - x} \right)}^2}\left( {2\frac{{\Delta x}}{{l - x}} + 1} \right) - \frac{1}{2}\frac{{{{\left( {l - x} \right)}^2}}}{{\Delta x}}{x^2}\left( {\frac{\beta }{{3D}}x + 1} \right)} \right]\frac{{{{\rm{d}}^2}{c_1}}}{{{\rm{d}}{t^2}}} + }\\ {\left[ {\left( {\beta + \beta \frac{x}{{\Delta x}} + \frac{D}{{\Delta x}}} \right){{\left( {l - x} \right)}^2}\left( {2\frac{{\Delta x}}{{l - x}} + 1} \right) - \frac{D}{{\Delta x}}{x^2}\left( {\frac{\beta }{{3D}}x + 1} \right)} \right]\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + }\\ {\left[ {\frac{D}{{\Delta x}}{x^2}\left( {\frac{\beta }{{3D}}x + 1} \right) - \left( {\frac{D}{{\Delta x}} + \beta \frac{x}{{\Delta x}}} \right){{\left( {l - x} \right)}^2}\left( {2\frac{{\Delta x}}{{l - x}} + 1} \right)} \right]\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} = 0。} \end{array} $ | (14) |
式(11)×D+式(12)×(D+βx),消去cl,1项得:
| $ \begin{array}{*{20}{c}} {\left[ {\frac{\beta }{2}{x^2} + \frac{\beta }{6}\frac{{{x^3}}}{{\Delta x}} + Dx + \frac{D}{2}\frac{{{x^2}}}{{\Delta x}} - \frac{1}{2}\frac{{{{\left( {l - x} \right)}^2}}}{{\Delta x}}\left( {D + \beta x} \right)} \right]\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + }\\ {\left\{ {\left[ {l - x + \frac{1}{2}\frac{{{{\left( {l - x} \right)}^2}}}{{\Delta x}}} \right]\left( {D + \beta x} \right) - \left( {\frac{\beta }{6}\frac{{{x^3}}}{{\Delta x}} + \frac{D}{2}\frac{{{x^2}}}{{\Delta x}}} \right)} \right\}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + \beta D{c_1} = \beta D{c^0}。} \end{array} $ | (15) |
式(14)+式(15)×2,消去
| $ \begin{array}{*{20}{c}} {\left[ {\left( {\frac{\beta }{{2D}}{x^2} + \frac{\beta }{{6D}}\frac{{{x^3}}}{{\Delta x}} + x + \frac{1}{2}\frac{{{x^2}}}{{\Delta x}}} \right){{\left( {l - x} \right)}^2}\left( {2\frac{{\Delta x}}{{l - x}} + 1} \right) - \frac{1}{2}\frac{{{{\left( {l - x} \right)}^2}}}{{\Delta x}}{x^2}\left( {\frac{\beta }{{3D}}x + 1} \right)} \right]\frac{{{{\rm{d}}^2}{c_1}}}{{{\rm{d}}{t^2}}} + }\\ {\left[ {\left( {\beta + \beta \frac{x}{{\Delta x}} + \frac{D}{{\Delta x}}} \right){{\left( {l - x} \right)}^2}\left( {2\frac{{\Delta x}}{{l - x}} + 1} \right) - \frac{D}{{\Delta x}}{x^2}\left( {\frac{\beta }{{3D}}x + 1} \right) + } \right.}\\ {\left. {\beta {x^2} + \frac{\beta }{3}\frac{{{x^3}}}{{\Delta x}} + 2Dx + D\frac{{{x^2}}}{{\Delta x}} - \frac{{{{\left( {l - x} \right)}^2}}}{{\Delta x}}\left( {D + \beta x} \right)} \right]\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + 2\beta D{c_1} = 2\beta D{c^0}。} \end{array} $ |
将上式的中括号内的乘式展开,合并项后,得:
| $ \begin{array}{*{20}{c}} {\left[ {\frac{\beta }{D}\Delta x\left( {l - x} \right){x^2} + \frac{\beta }{{3D}}{x^3}\left( {l - x} \right) + 2\left( {l - x} \right)x\Delta x + \left( {l - x} \right){x^2} + \frac{\beta }{{2D}}{x^2}{{\left( {l - x} \right)}^2} + x{{\left( {l - x} \right)}^2}} \right]\frac{{{{\rm{d}}^2}{c_1}}}{{{\rm{d}}{t^2}}} + }\\ {\left[ {2\beta \left( {l - x} \right)\Delta x + 2\beta x\left( {l - x} \right) + 2Dl + \beta {{\left( {l - x} \right)}^2} + \beta {x^2}} \right]\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + 2\beta D{c_1} = 2\beta D{c^0}。} \end{array} $ |
当Δx→0时,c1→cx,上式变为
| $ x\left( {l - x} \right)\left( {l + \frac{1}{2}lx\frac{\beta }{D} - \frac{1}{6}{x^2}\frac{\beta }{D}} \right)\frac{{{{\rm{d}}^2}{c_x}}}{{{\rm{d}}{t^2}}} + l\left( {l\beta + 2D} \right)\frac{{{\rm{d}}{c_x}}}{{{\rm{d}}t}} + 2\beta D{c_x} = 2\beta D{c^0}。$ | (16) |
将式(13) 的中括号内展开,得:
| $ \begin{array}{*{20}{c}} {\left[ {\frac{\beta }{D}{x^2}\left( {l - x} \right)\Delta x + \frac{\beta }{{3D}}{x^3}\left( {l - x} \right) + 2\left( {l - x} \right)x\Delta x + {x^2}\left( {l - x} \right) + \frac{\beta }{{2D}}{x^2}{{\left( {l - x} \right)}^2} + \frac{\beta }{{6D}}\frac{{{x^3}}}{{\Delta x}}{{\left( {l - x} \right)}^2} + } \right.}\\ {\left. {x{{\left( {l - x} \right)}^2} + \frac{1}{2}\frac{{{x^2}}}{{\Delta x}}{{\left( {l - x} \right)}^2} - \frac{\beta }{{6D}}\frac{{{{\left( {l - x} \right)}^2}}}{{\Delta x}}{x^3} - \frac{1}{2}\frac{{{{\left( {l - x} \right)}^2}}}{{\Delta x}}{x^2}} \right]\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + }\\ {\left[ {2\beta \left( {l - x} \right)\Delta x + 2\beta \left( {l - x} \right)x + 2\left( {l - x} \right)D + \beta {{\left( {l - x} \right)}^2} + \beta \frac{x}{{\Delta x}}{{\left( {l - x} \right)}^2} + \frac{D}{{\Delta x}}{{\left( {l - x} \right)}^2} - } \right.}\\ {\left. {\frac{\beta }{{3\Delta x}}{x^3} - \frac{D}{{\Delta x}}{x^2}} \right]{c_1} + \left[ {\frac{\beta }{{3\Delta x}}{x^3} + \frac{D}{{\Delta x}}{x^2} - 2\left( {l - x} \right)D - 2\beta \left( {l - x} \right)x - } \right.}\\ {\left. {\frac{D}{{\Delta x}}{{\left( {l - x} \right)}^2} - \beta \frac{x}{{\Delta x}}{{\left( {l - x} \right)}^2}} \right]{c_{l,1}} = \beta {c^0}{{\left( {l - x} \right)}^2}\left( {2\frac{{\Delta x}}{{l - x}} + 1} \right)。} \end{array} $ |
考虑到Δx为无穷小,上式化简并再整理为
| $ \begin{array}{*{20}{c}} {x\left( {l - x} \right)\left( {l + \frac{\beta }{{2D}}lx - \frac{\beta }{{6D}}{x^2}} \right)\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + \beta {{\left( {l - x} \right)}^2}{c_1} + \left[ {2\beta \left( {l - x} \right)x + 2\left( {l - x} \right)D} \right]\left( {{c_1} - {c_{l,1}}} \right) + }\\ {\left[ {\frac{\beta }{3}{x^3} + D{x^2} - \beta {{\left( {l - x} \right)}^2}x - D{{\left( {l - x} \right)}^2}} \right]\frac{{{c_{l,1}} - {c_1}}}{{\Delta x}} = \beta {{\left( {l - x} \right)}^2}{c^0}。} \end{array} $ |
当Δx→0时,c1→cx,cl,1→cx,
| $ \frac{{{\rm{d}}{c_x}}}{{{\rm{d}}t}} = \frac{{\beta \left( {l - x} \right)}}{{x\left( {l + \frac{\beta }{{2D}}lx - \frac{\beta }{{6D}}{x^2}} \right)}}\left( {{c^0} - {c_x}} \right) + \frac{{\frac{\beta }{3}{x^3} + D{x^2} - \beta {{\left( {l - x} \right)}^2}x - D{{\left( {l - x} \right)}^2}}}{{x\left( {l - x} \right)\left( {l + \frac{\beta }{{2D}}lx - \frac{\beta }{{6D}}{x^2}} \right)}} \times \frac{{{\rm{d}}{c_x}}}{{{\rm{d}}x}}\left| {_t} \right.。$ | (17) |
式(16) 是一个关于cx的二阶常系数线性微分方程。当0 < x < l时,其特征方程总有两个不相等且都小于零的实根r1、r2:
| $ {r_1} = \frac{{ - l\left( {l\beta + 2D} \right) - \sqrt {{l^2}{{\left( {l\beta + 2D} \right)}^2} - 8x\left( {l - x} \right)\left( {l + \frac{1}{2}lx\frac{\beta }{D} - \frac{1}{6}{x^2}\frac{\beta }{D}} \right)\beta D} }}{{2x\left( {l - x} \right)\left( {l + \frac{1}{2}lx\frac{\beta }{D} - \frac{1}{6}{x^2}\frac{\beta }{D}} \right)}}, $ |
| $ {r_2} = \frac{{ - l\left( {l\beta + 2D} \right) + \sqrt {{l^2}{{\left( {l\beta + 2D} \right)}^2} - 8x\left( {l - x} \right)\left( {l + \frac{1}{2}lx\frac{\beta }{D} - \frac{1}{6}{x^2}\frac{\beta }{D}} \right)\beta D} }}{{2x\left( {l - x} \right)\left( {l + \frac{1}{2}lx\frac{\beta }{D} - \frac{1}{6}{x^2}\frac{\beta }{D}} \right)}}。$ |
此微分方程的通解为
| $ {c_x} = {C_1}{{\rm{e}}^{{r_1}t}} + {C_2}{{\rm{e}}^{{r_2}t}} + {c^0}, $ | (18) |
在式(17) 中,令t=0得:
| $ \frac{{{\rm{d}}{c_x}}}{{{\rm{d}}t}}\left| {_{t = 0}} \right. = \frac{{\beta \left( {l - x} \right)}}{{x\left( {l + \frac{\beta }{{2D}}lx - \frac{\beta }{{6D}}{x^2}} \right)}}\left( {{c^0} - {c_x}\left| {_{t = 0}} \right.} \right) + \frac{{\frac{\beta }{3}{x^3} + D{x^2} - \beta {{\left( {l - x} \right)}^2}x - D{{\left( {l - x} \right)}^2}}}{{x\left( {l - x} \right)\left( {l + \frac{\beta }{{2D}}lx - \frac{\beta }{{6D}}{x^2}} \right)}} \cdot \frac{{{\rm{d}}{c_x}\left| {_{t = 0}} \right.}}{{{\rm{d}}x}}。$ |
而由方程(1) 的初始条件知:
| $ {c_x}\left| {_{t = 0}} \right. = 0, $ | (19) |
所以:
| $ \frac{{{\rm{d}}{c_x}}}{{{\rm{d}}t}}\left| {_{t = 0}} \right. = \frac{{\beta \left( {l - x} \right)}}{{x\left( {l + \frac{\beta }{{2D}}lx - \frac{\beta }{{6D}}{x^2}} \right)}}{c^0}。$ | (20) |
以式(19)、式(20) 作为边界条件确定式(18) 中的积分常数C1、C2,得cx(t)的一个近似式:
| $ {c_x} = - \left[ {{r_2} + \frac{{\beta \left( {l - x} \right)}}{{x\left( {l + \frac{\beta }{{2D}}lx - \frac{\beta }{{6D}}{x^2}} \right)}}} \right]\frac{{{c^0}}}{{{r_2} - {r_1}}}{{\rm{e}}^{{r_1}t}} + \left[ {{r_1} + \frac{{\beta \left( {l - x} \right)}}{{x\left( {l + \frac{\beta }{{2D}}lx - \frac{\beta }{{6D}}{x^2}} \right)}}} \right]\frac{{{c^0}}}{{{r_2} - {r_1}}}{{\rm{e}}^{{r_2}t}} + {c^0}。$ | (21) |
反应器内团块、气泡、粉粒或液滴内的扩散,层状液、液界面两侧内扩散都是有限长度区间上的传质问题;获得有限长度区间上扩散方程的近似分析解,兼有实践意义和理论意义。首先对动力学研究中稳态近似法的原理进行了分析,并将经常应用的浓度随时间不变的稳态假设发展为浓度变化率随时间不变的稳态假设。以质量守衡原理、菲克扩散定律为物理基础,以对方程的离散化处理为手段,以浓度变化率随时间不变为稳态约束,可获得各步骤扩散速率的近似表达式;以扩散速率稳态近似式的利用为核心,给出了对有限长度区间内扩散方程进行稳态近似法处理的过程;同时获得了二、三类边界条件下扩散方程的一个稳态近似解。对近似解和精确解的偏差来源进行了分析。对稳态近似解进行了分析,并与准确度很高的数值解做了比较,所得结果在另文中予以叙述。
附录:
由式(2-1) 变形得:
| $ {c_2} = {c_1} + \frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_1} - \frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_{l,1}} + \frac{1}{2}\frac{1}{D}\left( {\frac{{x - 0.5\Delta x}}{{n - 1}} + \Delta x} \right)\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}}, $ |
将c2对时间求导,并结合假设得:
| $ \frac{{{\rm{d}}{c_2}}}{{{\rm{d}}t}} = \frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + \frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - \frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}}, $ |
由式(2-2) 变形得:
将
| $ \begin{array}{*{20}{c}} {{c_3} = {{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^2}\frac{1}{D}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - {{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + }\\ {\frac{1}{D}\left( {\frac{{x - 0.5\Delta x}}{{n - 1}} + \Delta x} \right)\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {c_1} + 2\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_1} - 2\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_{l,1}}。} \end{array} $ |
将c3对时间求导,并结合假设得:
| $ \frac{{{\rm{d}}{c_3}}}{{{\rm{d}}t}} = \frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + 2\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - 2\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}}, $ |
由式(2-3) 变形得:
将
| $ \begin{array}{*{20}{c}} {{c_4} = 3{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^2}\frac{1}{D}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + 4{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - 4{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + }\\ {\frac{3}{2}\frac{1}{D}\left( {\frac{{x - 0.5\Delta x}}{{n - 1}} + \Delta x} \right)\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {c_1} + 3\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_1} - 3\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_{l,1}}} \end{array} $ |
综合c3和c4表达式的形式,令:
| $ \begin{array}{*{20}{c}} {{c_i} = {A_i}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^2}\frac{1}{D}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {B_i}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - {B_i}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + }\\ {\frac{{{C_i}}}{2}\frac{1}{D}\left( {\frac{{x - 0.5\Delta x}}{{n - 1}} + \Delta x} \right)\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {c_1} + {C_i}\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_1} - {C_i}\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_{l,1}}。} \end{array} $ | (4) |
则:
| $ \begin{array}{*{20}{c}} {{c_{i - 1}} = {A_{i - 1}}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^2}\frac{1}{D}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {B_{i - 1}}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - {B_{i - 1}}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + }\\ {\frac{{{C_{i - 1}}}}{2}\frac{1}{D}\left( {\frac{{x - 0.5\Delta x}}{{n - 1}} + \Delta x} \right)\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {c_1} + {C_{i - 1}}\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_1} - {C_{i - 1}}\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_{l,1}};} \end{array} $ |
| $ \begin{array}{*{20}{c}} {{c_{i - 2}} = {A_{i - 2}}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^2}\frac{1}{D}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {B_{i - 2}}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - {B_{i - 2}}{{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + }\\ {\frac{{{C_{i - 2}}}}{2}\frac{1}{D}\left( {\frac{{x - 0.5\Delta x}}{{n - 1}} + \Delta x} \right)\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {c_1} + {C_{i - 2}}\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_1} - {C_{i - 2}}\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_{l,1}}。} \end{array} $ |
将ci-1对时间求导,并结合假设得:
| $ \frac{{{\rm{d}}{c_{i - 1}}}}{{{\rm{d}}t}} = \frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + {C_{i - 1}}\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - {C_{i - 1}}\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}}。$ |
由式[2-(i-1)]得:
将
| $ \begin{array}{*{20}{c}} {{c_i}=\left( {1 + 2{A_{i - 1}} - {A_{i - 2}}} \right){{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^2}\frac{1}{D}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + \left( {2{B_{i - 1}} - {B_{i - 2}} + {C_{i - 1}}} \right){{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} - }\\ {\left( {2{B_{i - 1}} - {B_{i - 2}} + {C_{i - 1}}} \right){{\left( {\frac{{x - 0.5\Delta x}}{{n - 1}}} \right)}^3}\frac{1}{D}\frac{1}{{\Delta x}}\frac{{{\rm{d}}{c_{l,1}}}}{{{\rm{d}}t}} + \frac{{\left( {2{C_{i - 1}} - {C_{i - 2}}} \right)}}{2}\frac{1}{D}\left( {\frac{{x - 0.5\Delta x}}{{n - 1}} + \Delta x} \right)\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{{{\rm{d}}{c_1}}}{{{\rm{d}}t}} + }\\ {{c_1} + \left( {2{C_{i - 1}} - {C_{i - 2}}} \right)\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_1} - \left( {2{C_{i - 1}} - {C_{i - 2}}} \right)\frac{{x - 0.5\Delta x}}{{n - 1}}\frac{1}{{\Delta x}}{c_{l,1}}。} \end{array} $ |
将此式和式(4) 比照,得Ai、Bi和Ci的递推公式(i≥5):Ai=2Ai-1-Ai-2+1,A3=1,A4=3;Bi=2Bi-1-Bi-2+Ci-1,B3=1,B4=4;Ci=2Ci-1-Ci-2,C3=2,C4=3。A3、A4、B3、B4、C3、C4是c3、c4表达式中相应项前面的系数。由Ai、Bi和Ci的递推公式及相应前两项的值可推得:
| [1] | 黄希祜. 钢铁冶金原理[M]. 3版. 北京: 冶金工业出版社, 2002. |
| [2] | 肖兴国. 冶金反应工程学[M]. 沈阳: 东北工学院出版社, 1989. |
| [3] | 韩其勇. 冶金过程动力学[M]. 北京: 冶金工业出版社, 1983. |
| [4] | Bonalde A, Henriquez A, Manrique M. Kinetic analysis of the iron oxide reduction using hydrogen-carbon monoxide mixtures as reducing agent[J]. ISIJ International, 2005, 45(9): 1255–1260. DOI:10.2355/isijinternational.45.1255 |
| [5] | 肖兴国, 谢蕴国. 冶金反应工程学基础[M]. 北京: 冶金工业出版社, 1997. |
| [6] | Sohn H Y, Perez-Fontes S E. Application of the law of additive reaction times to fluid-solid reactions in porous pellets with changing effective diffusivity[J]. Metallurgical and Materials Transactions B, 2010, 41(6): 1261–1267. DOI:10.1007/s11663-010-9414-0 |
| [7] | Sohn H Y. The influence of chemical equilibrium on fluid-solid reaction rates and the falsification of activation energy[J]. Metallurgical and Materials Transactions B, 2004, 35(1): 121–131. DOI:10.1007/s11663-004-0102-9 |
| [8] | Sohn H Y. The effects of reactant starvation and mass transfer in the rate measurement of fluid-solid reactions with small equilibrium constants[J]. Chemical Engineering Science, 2004, 59(20): 4361–4368. DOI:10.1016/j.ces.2004.06.033 |
| [9] | Wu S L, Xu J, Yang S D, et al. Basic characteristics of the shaft furnace of COREX© smelting reduction process based on iron oxides reduction simulation[J]. ISIJ International, 2010, 50(7): 1032–1039. DOI:10.2355/isijinternational.50.1032 |
| [10] | Yang K, Choi S, Chung J, et al. Numerical modeling of reaction and flow characteristics in a blast furnace with consideration of layered burden[J]. ISIJ International, 2010, 50(7): 972–980. DOI:10.2355/isijinternational.50.972 |
| [11] | Dong X F, Yu A B, Yagi J I, et al. Modelling of multiphase flow in a blast furnace:recent developments and future work[J]. ISIJ International, 2007, 47(11): 1553–1570. DOI:10.2355/isijinternational.47.1553 |
| [12] | Chuang H C, Kuo J H, Huang C C, et al. Multi-phase flow simulations in direct iron ore smelting reduction process[J]. ISIJ International, 2006, 46(8): 1158–1164. DOI:10.2355/isijinternational.46.1158 |
| [13] | Chu M S, Nogami H, Yagi J I. Numerical analysis on blast furnace performance under operation with top gas recycling and carbon composite agglomerates charging[J]. ISIJ International, 2004, 44(12): 2159–2167. DOI:10.2355/isijinternational.44.2159 |
| [14] | 沈颐身, 李保卫, 吴懋林. 冶金传输原理基础[M]. 北京: 冶金工业出版社, 2000. |
| [15] | Coulson J M, Richardson J F. Chemical engineering:fluid flow, heat transfer and mass transfer[M]. 6th ed.Wellington: Butterworth-Heinemann, 1999. |
| [16] |
王成善, 黄太镭, 穆小静, 等.
二、三类边界条件下扩散方程的稳态近似分析[J]. 重庆大学学报, 2013, 36(9): 118–126.
WANG Chengshan, HUANG Tailei, MU Xiaojing, et al. Analysis of diffusion equation with the second and third boundary conditions based on steady state approximation[J]. Journal of Chongqing university, 2013, 36(9): 118–126. DOI:10.11835/j.issn.1000-582X.2013.09.019 (in Chinese) |
| [17] | 王淑兰. 物理化学[M]. 3版. 北京: 冶金工业出版社, 2008. |
| [18] | 梁英教. 物理化学[M]. 北京: 冶金工业出版社, 2003. |
| [19] | 陶文铨. 数值传热学[M]. 西安: 西安交通大学出版社, 1995. |
 2014, Vol. 37
2014, Vol. 37


